
Image Purchased by Passy World from Dreamstime.com
In this lesson we are looking at Interest Money associated with Loans and Investments.
When money is Borrowed or Invested, INTEREST is paid – to the Investor – or – by the Borrower.
We will only be looking at “Simple Interest” where the money is paid out at regular times, and is not left to “compound” and grow.
This means that the Interest does not earn any extra interest on itself, it is simply generated one time only.
Just to repeat, this lesson is on “Simple Interest” and does not teach “Compound Interest”.
In addition, this lesson only covers Interest being paid Yearly.
We will be covering Half-Yearly, Quarterly, Monthly, and Daily Interest payment in our “Simple Interest – Part II” lesson.
Video About Simple Interest
The following six minute Discovery Channel video gives an introduction to Simple Interest.
Simple Interest Formula
The following Mathematical Formula is used for calculating “Simple Interest”, (symbol “I”).
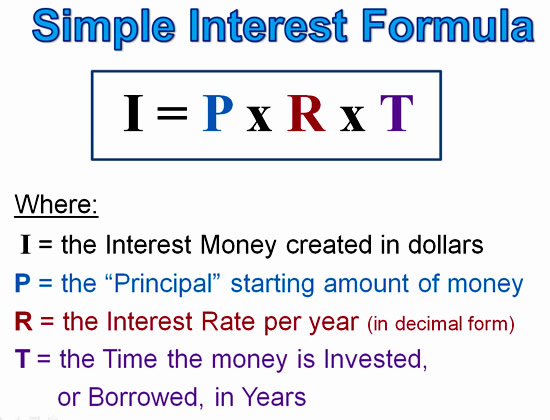
Image Copyright 2013 by Passy’s World of Mathematics
Let’s go through these variable letters in the formula one by one in more detail.
The “P” Principal

Image Source: http://www.lebeauty.com.au
The Principal is the original amount of money invested, or borrowed.
For example, if Jodie gets a $5000 loan for a car, then the “Principal” is
P = 5000.
And if Luke invests $2000 at 3% Interest per annum for three years, then the Principal is P = 2000.
The “R” Interest Rate

Image Source: http://resources2.news.com.au
If you have a lot of borrowed money, like for a housing mortgage, then even a small change in Interest Rate will cause a significant financial burden.
This happens because a small percent like 0.5%, of a big number like a mortgage for $400 000 will require the owner to pay an extra $2000 Interest in the next 12 months.
Percent Rates usually have a “pa” after them, such as 3% pa or 16% pa.
The “pa” is a short hand form for “per annum”, which means “per year”.
10% pa means that each year there is 10% of the Principal as extra Interest money created.
For the I = PRT formula, the “R” must be converted to a decimal value before putting R into the formula.
The Percent value of R is converted to a decimal by dividing by 100.
The “T” Time Value

Image Purchased by Passy World from Dreamstime.com
Over time the original “Principal” amount of money grows as Interest is added each year. Unfortunately this growth rate is in Years, rather than in hours and minutes.
The Time value in the I = PRT formula must always be entered in years.
Howver not all Interest payments are organised as Yearly. This adds a little bit of complication which involves the use of fractions.
Interest can be calculated to be paid Half-Yearly, Quarterly, Monthly, or even Daily.
For these situations, “T” needs to be expressed as a fraction of a year because the Interest Rate will usually be an Annual yearly Rate.
This is shown in the diagram below.
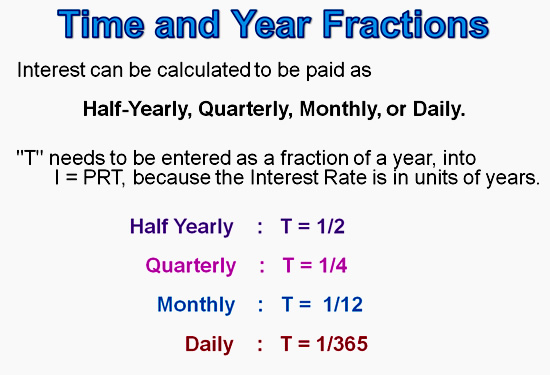
Image Copyright 2013 by Passy’s World of Mathematics
We will be covering Half-Yearly, Quarterly, Monthly, and Daily Interest payment in our “Simple Interest – Part II” lesson.
The Interest Rate on a Loan may not seem to be all that high, (eg. less than 20%).
However it is very important to realise how this Rate multiplied by the Time in the I = PRT formula leads to much higher than expected sums of money being involved.
This is explained in the following two minute video.
Video About Doing Simple Interest Calculations
The following fourteen minute video shows how to do common Simple Interest Calculations.
Example 1 – Calculating Interest

Image Purchased by Passy World from Dreamstime.com
“Roxanna borrows some money to buy a new car.
The loan is for $6000 at 18.5% pa Simple Interest, to be paid back over 5 years.
Calculate the amount of Interest she will have paid by the end of the 5 years.”
We need to use I = PRT to answer this question. But the “R” value of 18.5% needs to be converted to a Decimal by doing 18.5 divided by 100 to get 0.185
The working out is shown below.
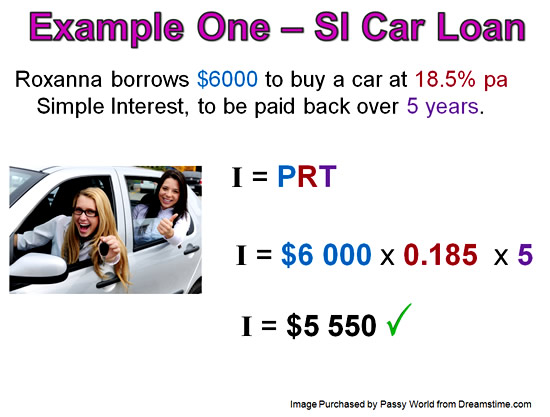
Image Copyright 2013 by Passy’s World of Mathematics
The Total Amount Roxanna has to repay is all of the $5550 Interest, plus the original $6000.
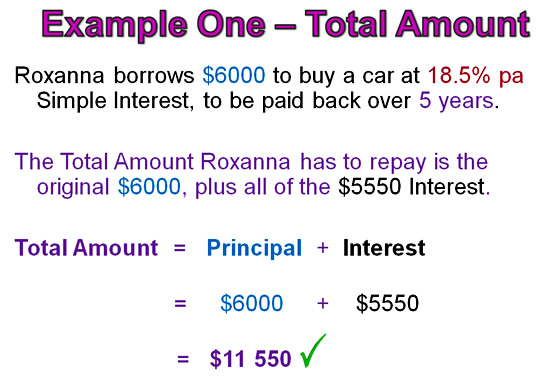
Image Copyright 2013 by Passy’s World of Mathematics
Example 2 – Calculating Time

Image Source: http://www.johnkapili.com
Excel Spreadsheets with the I = PRT formula written into them can be used for doing Simple Interest Calculations.
However, we will not be working out our answers with Excel. We will use good old fashioned pen and paper.
In some situations we need to calculate the Time that money needs to be invested to earn a certain amount of Interest.
We can use one of two methods:
Method 1 – Use I = PRT, substitute in the known values, and then solve the resulting Algebra Equation for T.
This was method was demonstrated in a previous Video in this lesson.
Method 2 – Rearrange I = PRT to become the T = I / (RxT) formula and use this “T” formula directly.
We suggest trying hard to use Method 1, as this will help consolidate Algebra Equations.
However if Method 1 is too difficult, then use Method 2.
Our calculating Time example is as follows:
“How long does Keith need to invest $4000 at 3.33% pa, to earn $800 of Interest money?”
We have used Method 2 in the working out shown below.
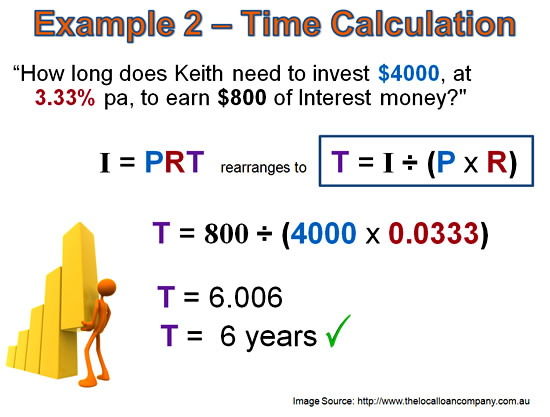
Image Copyright 2013 by Passy’s World of Mathematics
Example 3 – Calculating Rate

Image Source: http://sklepcukrzyka.pl
To calculate an unknown Rate, we can use one of two methods:
Method 1 – Use I = PRT, substitute in the known values, and then solve the resulting Algebra Equation for R.
This was method was demonstrated in a previous Video in this lesson.
Method 2 – Rearrange I = PRT to become the R = I / (PxT) formula and use this “R” formula directly.
We suggest trying hard to use Method 1, as this will help consolidate Algebra Equations.
However if Method 1 is too difficult, then use Method 2.
Our calculating Rate example is as follows:
“What Interest Rate do we require to get $10 000 of interest money generated from investing $20 000 for 3 years ?”
We have used Method 2 in the working out shown below.
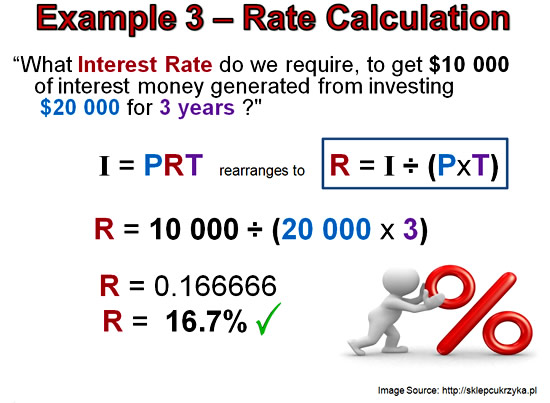
Image Copyright 2013 by Passy’s World of Mathematics
Example 4 – Calculating Principal

Image Source: http://static.guim.co.uk
In this example we are finding the Principal Amount.
“Tayla borrowed money on 22% Simple Interest for 1 year to pay for some expensive Christmas Presents.
When she payed back the Loan a year later, the Interest that was charged was $440.
What was the original Principal Amount she borrowed ?”
The solution (using Method 2 – Direct Formula) is shown below.
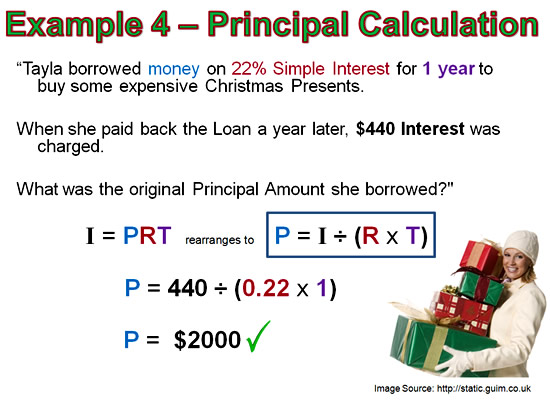
Image Copyright 2013 by Passy’s World of Mathematics
More Videos on Simple Interest
Here are a few more videos that show examples of “Simple Interest” calculations.
Subscribe
If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.
If you are a subscriber to Passy’s World of Mathematics, and would like to receive a free PowerPoint version of this lesson, that is 100% free to you as a Subscriber, then email us at the following address:
Please state in your email that you wish to obtain the free subscriber copy of the “Simple Interest One” Powerpoint.
Help Passy’s World Grow
Each day Passy’s World provides hundreds of people with mathematics lessons free of charge.
Help us to maintain this free service and keep it growing.
Donate any amount from $2 upwards through PayPal by clicking the PayPal image below. Thank you!
PayPal does accept Credit Cards, but you will have to supply an email address and password so that PayPal can create a PayPal account for you to process the transaction through. There will be no processing fee charged to you by this action, as PayPal deducts a fee from your donation before it reaches Passy’s World.
Enjoy,
Passy





Pingback: Simple Interest – Part 2 | Passy's World of Mathematics