Image Source: http://i.telegraph.co.uk
In this lesson we look at Six Monthly, Quarterly, Monthly, and Daily Simple Interest.
In particular we look at Simple Interest Calculated on Bank Accounts.
It is recommended that you have done our previous Part 1 lesson, (at the link below), before attempting this lesson.
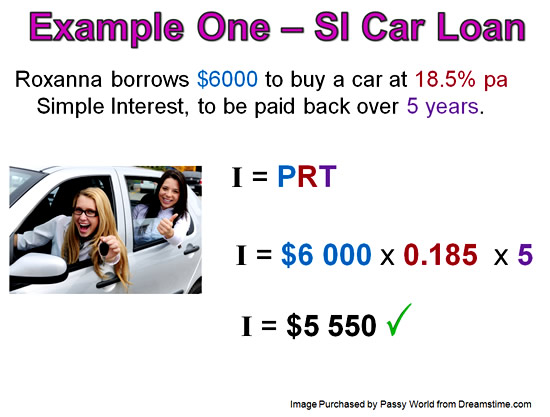
Basic Simple Interest Calculations
Simple Interest Formula
The following is the mathematical formula we use for all Simple Interest calculations:
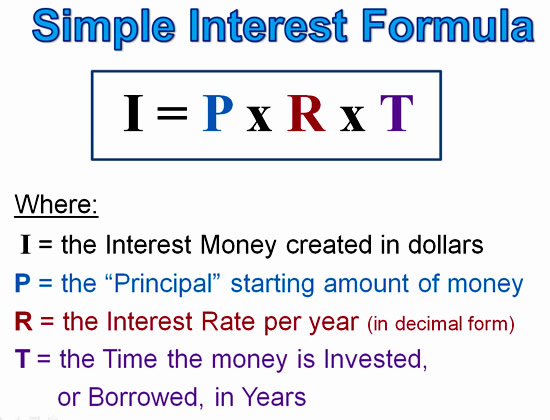
Image Copyright 2013 by Passy’s World of Mathematics
When Six Monthly (or “Half Yearly”), Quarterly, Monthly, or Daily Simple Interest are involved we need to use the fractions shown below.
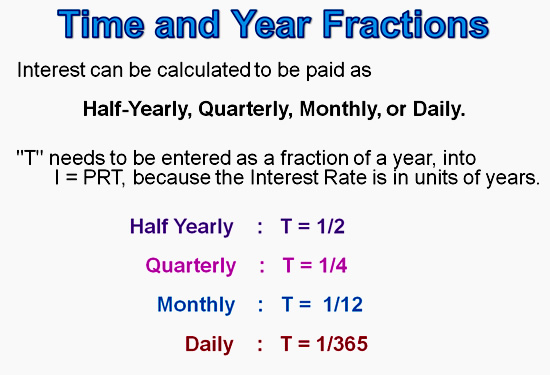
Image Copyright 2013 by Passy’s World of Mathematics
This is because Time must always be in Years when we use the I = PRT formula.
Example One – Half Yearly Interest

Image Source: http://www.toogezer.com
Tahli borrows $2000 to buy a new TV at 18.5% pa Simple Interest charged 6 monthly, to be paid back over 2 years.
In this example Interest is calculated twice a year, or every six months.
This means the first thing we have to do is calculate how many dollars there are in one lot of six monthly interest.
The calculation is as shown below.

Image Copyright 2013 by Passy’s World of Mathematics
Now that we know what the Interest is every 6 months, all we need to do is work out how many lots of six months there are in the loan term of 2 years.
We can then multiply out and calculate the Total Interest to be paid over the loan as shown below.

Image Copyright 2013 by Passy’s World of Mathematics
Note that we can also calculate this same Total Interest value, using I = PRT and substitute the total time of 2 years:
I = PRT = 2000 x 0.185 x 2 = $740
It is an excellent idea to do this quick calculation as a check of the work we have done getting our previous answer of $740
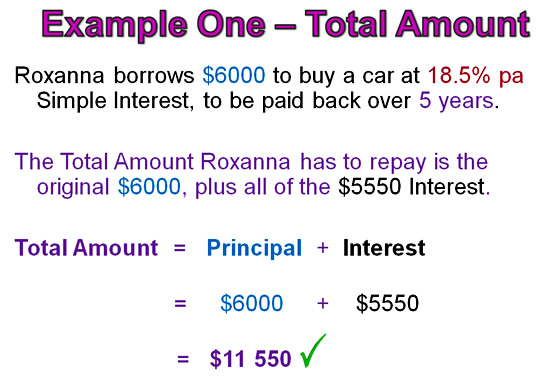
The Total Amount to be paid back is always the Total Interest plus the original Loan Amount:

Image Copyright 2013 by Passy’s World of Mathematics
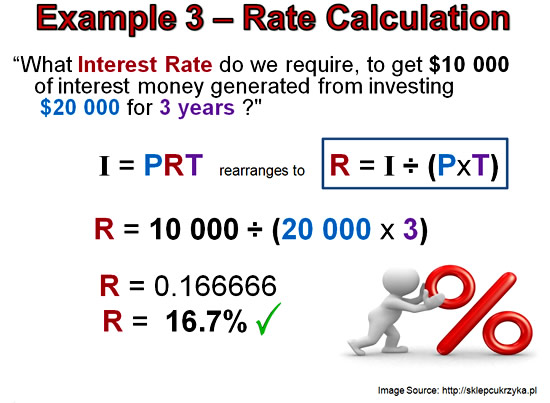
Example Two – Quarterly Interest

Image Purchased by Passy’s World from Dreamstime.com
Alexandra borrows $20 000 to set up a Beautician business at 16% pa Simple Interest charged Quarterly, to be paid back over 5 years and 3 months.
Because the Interest is paid Quarterly, the first step is to calculate the Interest each Quarter as shown below:

Image Copyright 2013 by Passy’s World of Mathematics
The next step is to now multiply out the Quarterly Interest over the full term of the Loan.
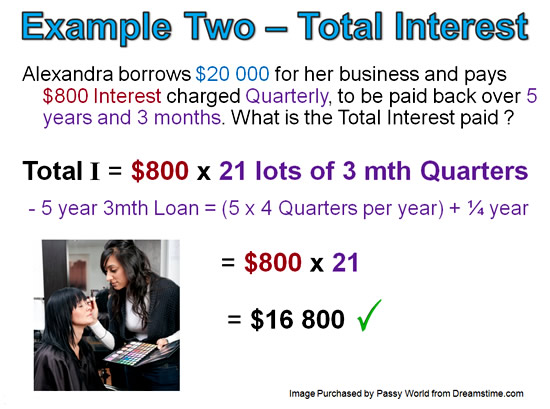
Image Copyright 2013 by Passy’s World of Mathematics
Finally we calculate the Total Cost of the Loan by adding together the Total Interest plus the original Loan Amount.

Image Copyright 2013 by Passy’s World of Mathematics
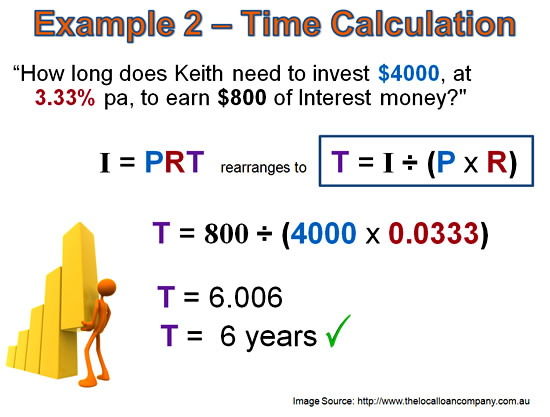
Example Three – Monthly Interest

Image Source: http://www.mutualsavings.org
Joyce Deposits $2 000 into an Internet Saver Account that gives her 5.4% pa Interest paid Monthly.
She leaves the money there for 2 ½ years.
Because the Interest is paid Monthly, the first step is to calculate the Interest for one Month as shown below:
( There are 12 months in a year, so one month = 1/12 th of a Year. )

Image Copyright 2013 by Passy’s World of Mathematics
We now need to work out how many Months there are in the 2 and one half year loan.
Each year = 12 months, and one half of a year = 6 months.
So in 2 and 1/2 years we have 12 months + 12 months + 6 months = 30 months,
We now multiply this 30 months x the Interest for one month, as shown below:

Image Copyright 2013 by Passy’s World of Mathematics
Finally we calculate the Total Cost of the Loan by adding together the Total Interest plus the original Loan Amount.

Image Copyright 2013 by Passy’s World of Mathematics
Example 4 – Minimum Monthly Balance

Image Source: http://www.bankofscotlandbusiness.co.uk
Some Bank Accounts give Daily Interest fr the total days in a month, by taking the Account’s lowest monthly balance as the Principal $ value to use in I = PRT
The following example shows a very simple Bank Statement:

Image Copyright 2013 by Passy’s World of Mathematics
Once we have located the Minimum Balance value, and realise that June is a 30 day month, the Interest Calculation is quite straight forward:
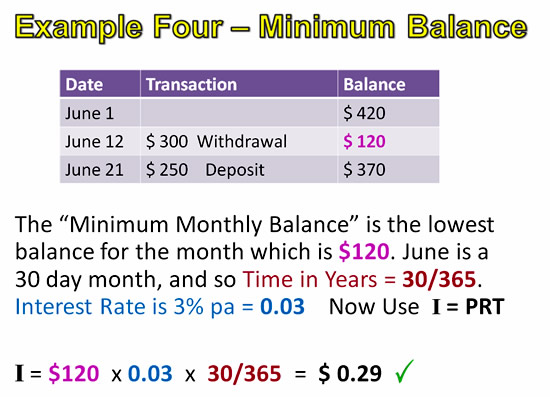
Image Copyright 2013 by Passy’s World of Mathematics
Example 5 – Daily Interest on Bank Accounts

Image Source: http://webmedia.bankofamerica.com
In the previous example we saw how Bank Interest is calculated on some Bank Account using the “Minimum Monthly Balance” method.
It is much better to have a Bank Account which calculates Interest based on the Daily Balance in the account. This will type of account will pay far more interest money than a Minimum Monthly Balance account.
Calculating Daily Interest that is paid at the end of each month is a bit more complicated as shown in the example below.

Image Copyright 2013 by Passy’s World of Mathematics
The first step is to add a “Number of Days” Column onto our table, and work out how many days the account was at each of the balances.

Image Copyright 2013 by Passy’s World of Mathematics
Once we have our Days column worked out, we can calculate the I = PRT interest for each of the balances, and then add them up to get the total interest for the month.
This is shown below:

Image Copyright 2013 by Passy’s World of Mathematics
Compound Interest

Image Source: http://www.batr.org
Although not covered in this lesson, Compound Interest is the most powerful way of getting money to grow in value.
With Compound Interest, the Interest money is left in the account to also earn extra ongoing interest.
Unlike Simple Interest where the interest money is paid out at the end of each accumulation.
Here is a video about the Power of Compounding Interest.
Related Items
Basic Simple Interest Calculations
Subscribe
If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.
If you are a subscriber to Passy’s World of Mathematics, and would like to receive a free PowerPoint version of this lesson, that is 100% free to you as a Subscriber, then email us at the following address:
Please state in your email that you wish to obtain the free subscriber copy of the “Simple Interest Two” Powerpoint.
Help Passy’s World Grow
Each day Passy’s World provides hundreds of people with mathematics lessons free of charge.
Help us to maintain this free service and keep it growing.
Donate any amount from $2 upwards through PayPal by clicking the PayPal image below. Thank you!
PayPal does accept Credit Cards, but you will have to supply an email address and password so that PayPal can create a PayPal account for you to process the transaction through. There will be no processing fee charged to you by this action, as PayPal deducts a fee from your donation before it reaches Passy’s World.
Enjoy,
Passy