The answer to this question is quite simple:

Image Copyright 2013 by Passy’s World of Mathematics
Looking at the two photos, it is quite easy for a human to work out which person is in both pictures.
But imagine the Maths that would be needed, (coupled with some facial recognition software), for a computerised device to work this out.
Actually the program required is not too complicated. It could use a Database of Facial Images, a lookup table, and some mathematics in the form of Sort/Match array processing code.
But this is turning into a High School IT lesson, so let’s get back to the Maths!
In this lesson we will be looking at Algebra Terms in expressions, and working out if the separate terms have anything in common.
Once we have worked out the “Common Factor”, it is then a fairly simple task to rewrite our original expression in “Factor Form”, (eg. a Form which contains brackets).
Prime Factors and Factor Trees
Before we can do the Common Factor work for this lesson, we first need to review “Prime Factors”.

Image Copyright 2013 by Passy’s World of Mathematics
In the images shown here we are building “Factor Trees” to work out the Prime Factors.
The Tree shapes are actually upside down in these diagrams, and the ends of each branch are called the “leaves” and this is where our Prime Number factors end up.
We combine together the leaves on the ends of the branches, and this gives us our set of Prime Factors.

Image Copyright 2012 by Passy’s World
Watch the videos in the following section if you do not already know how to make “Prime Factors” for numbers.
Prime Factors Videos
And finally watch this great music song video from “Math Rocks!”
Expanded Form and Factor Form
When we expanded brackets using the Distributive Rule, we created an answer containing no brackets which is called “Expanded Form”.
Expanded Form is useful when we wnat to solve an Equation, or draw a Graph on an X-Y Grid.
If you need to review expanding brackets, then check out our previous lesson at the link below:
http://passyworldofmathematics.com/expanding-brackets-using-distributive-rule/
In this lesson we are doing the reverse process of Expanding, which is called “Factorisation”.
Factorising results in us getting an Expression which contains brackets.
Factorised Expressions are used for locating the horizontal X-Axis cross-over points on Graphs.
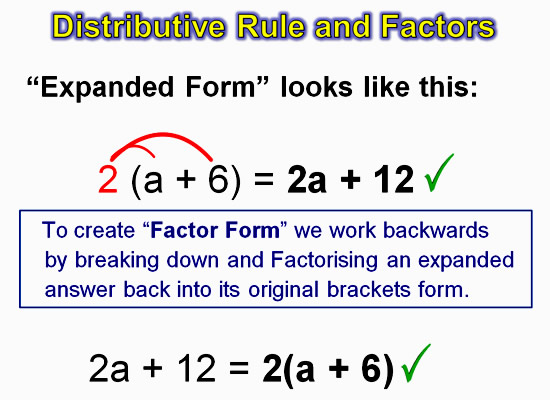
Image Copyright 2013 by Passy’s World of Mathematics
Factorising Steps
The steps we follow to convert an Expanded expression into a Factorised expression are as follows.

Image Copyright 2013 by Passy’s World of Mathematics
Common Factor Examples
The following examples show how we do Factorisation to create answers that have a single bracket and are in Factor Form.
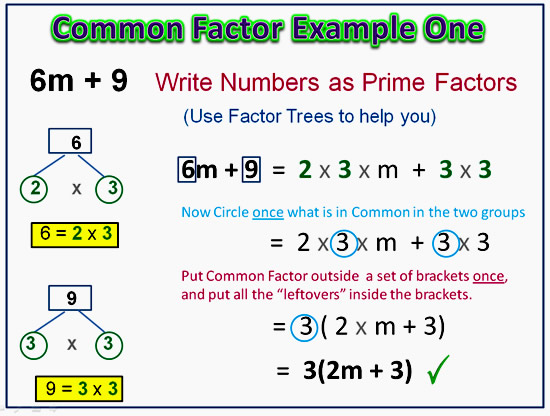
Image Copyright 2013 by Passy’s World of Mathematics

Image Copyright 2013 by Passy’s World of Mathematics
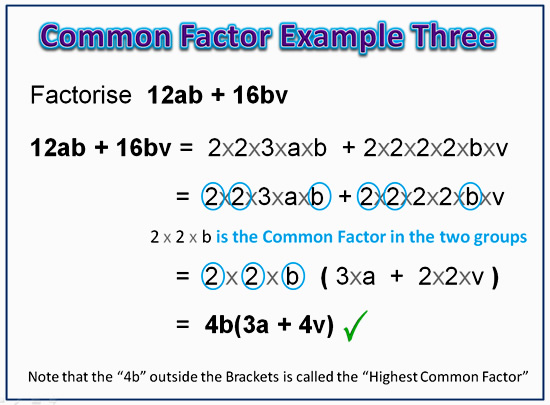
Image Copyright 2013 by Passy’s World of Mathematics
Common Factors Involving Exponents
When our initial terms contain variable letters and exponents, we need to expand out the exponents, and then see what letters we have in common.
The following examples show this; where numbers and letters are both broken down as low as they can go, and then the common factors identified.

Image Copyright 2013 by Passy’s World of Mathematics

Image Copyright 2013 by Passy’s World of Mathematics
Common Factors Involving a Negative Sign
In the example below, we have a negative sign on both items, which means the negative sign is a Common Factor.
We write the negative sign as a -1 when we include it in the expanded factors.
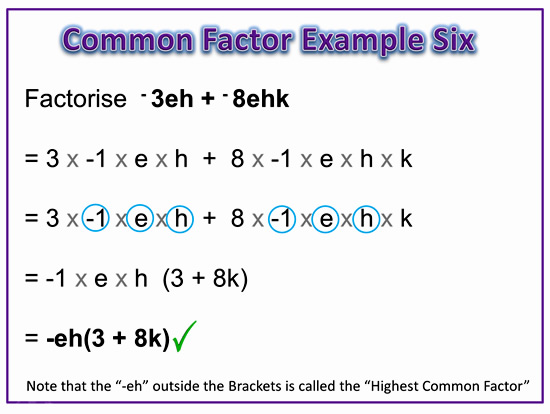
Image Copyright 2013 by Passy’s World of Mathematics
Videos About Factorising
The following video shows how to find the Highest Common Factor (HCF), or Greatest Common Factor (GCF), using Factor Trees.
This next video shows how to do the greatest common factor a faster way than using Prime Factors.
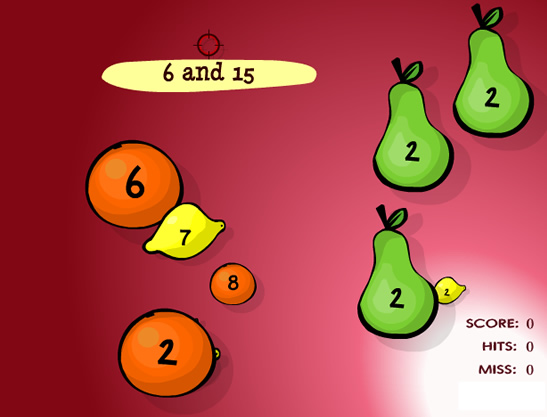
Common Factor Games
“Fruit Shoot” is a fun game where you have to work out what the biggest Common Factor is, and then use the mouse to move onto the correct piece of fruit and click on it.
Click the following link to play this fun game:
http://www.sheppardsoftware.com/mathgames/fractions/GreatestCommonFactor.htm
In this next Game, we slide the bottom pink boxes to their correct position on the question grid.
Click the following link to play this fun game:
http://www.oswego.org/ocsd-web/match/dragflip.asp?filename=slanegcf
Related Items
Expanding Single Brackets
Dividing Algebra Terms
Binomial Expansion
Subscribe
If you enjoyed this lesson, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our website, then please email us at the hotmail address shown in the right hand side bar of this page.
If you are a subscriber to Passy’s World of Mathematics, and would like to receive a free PowerPoint version of this lesson, that is 100% free to you as a Subscriber, then email us at the following address:
Please state in your email that you wish to obtain the free subscriber copy of the “Common Factors” Powerpoint.
Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.
Like Us on Facebook
Help Passy’s World Grow
Each day Passy’s World provides hundreds of people with mathematics lessons free of charge.
Help us to maintain this free service and keep it growing.
Donate any amount from $2 upwards through PayPal by clicking the PayPal image below. Thank you!
PayPal does accept Credit Cards, but you will have to supply an email address and password so that PayPal can create a PayPal account for you to process the transaction through. There will be no processing fee charged to you by this action, as PayPal deducts a fee from your donation before it reaches Passy’s World.
Enjoy,
Passy