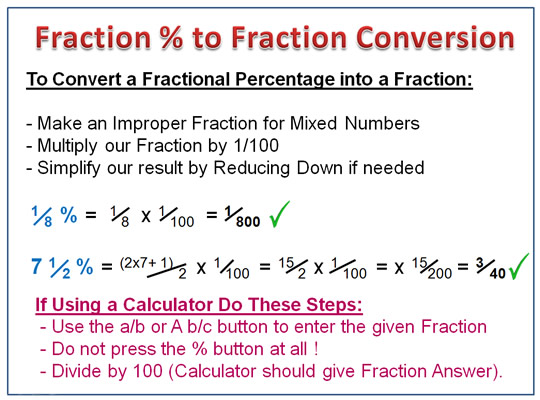
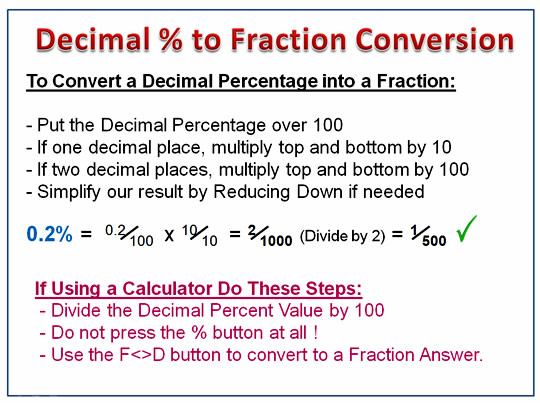
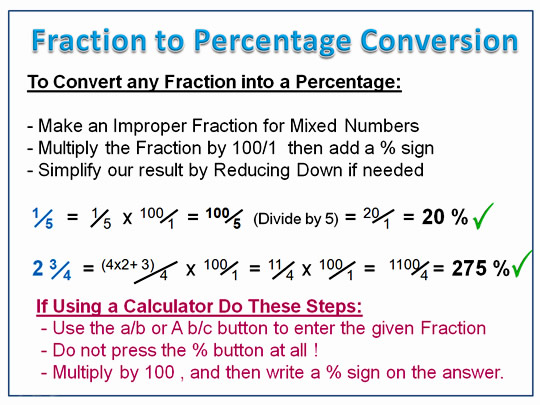
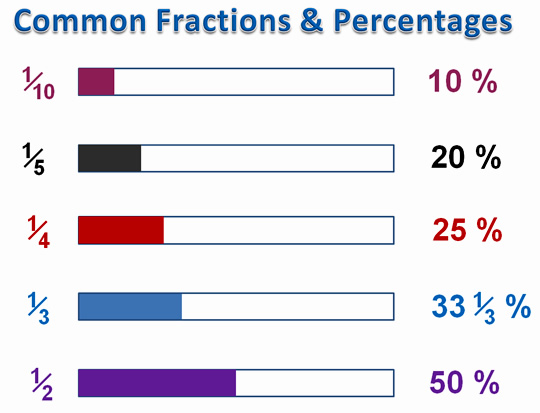
Image Source: http://resources0.news.com.au
In this lesson we look at some Mathematics related to the Olympic Games.
Marathon Distance Compared to Middle Distance
How long is the 800m middle distance running race in the Olympics, compared to the Marathon Race ?
We will answer this by comparing the 800m to the Marathon as a Percentage.
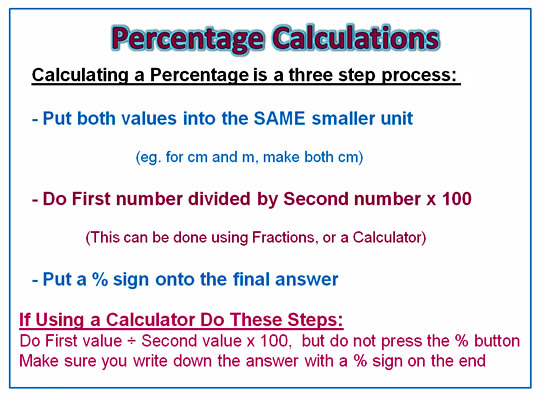
When we compare items and calculate percents, it is important that the items are both in the same units.
The Marathon is a long-distance running event with an official distance of 42.195 kilometres (26 miles and 385 yards), that is usually run as a road race.
The event was instituted in commemoration of the fabled run of the Greek soldier Pheidippides, a messenger who ran from the Battle of Marathon to Athens.
So we have:
800m Running Race compared to
42.195 km Marathon.
Some people will immediately do the calculation as follows:
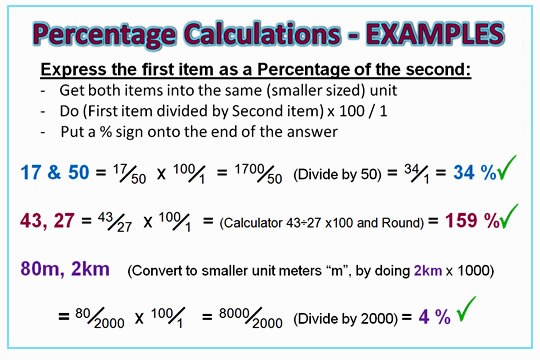
( 800 / 42.195 ) X ( 100/1 ) = 1896 %
This Percentage answer is way too big and makes it appear that an 800m race is much bigger than a 42 km Marathon!
We need to convert units before working out the Percentage !
For 800m and 42.195km the smaller unit is meters “m”, because a meter is much shorter than a whole km.
Each km = 1000m, and so we multiply the km by 1000
Eg. 42.195 x 1000 = 42 195 m.
So we work out our Percentage as follows:
% = ( 800 / 42195 ) X ( 100/1 )
% = 1.8959592
% = 2 %
Since 2% = 2/100 = one fiftieth, this means we would need to do fifty 800m runs to reach the distance of the Marathon.
Each 800m race is 2 laps of the running track, and so the Marathon is about 100 laps of the track.
If you are in Australia, then think about running around a football oval 100 times. This will give you an idea of how long the Marathon is.
The Marathon is a LONG race !
How Gold is a Gold Medal ?

Image Source: http://media.nowpublic.net
The incredible Michael Phelps from the USA won eight gold swimming medals in the one Olympics.
But how much Gold is in a Gold Medal ?
Gold Medals weigh 500 grams. Only 6 grams of this is Pure Gold, the rest is usually Silver metal.
As a percentage, for Gold we have:
( 6 / 500 ) x ( 100 / 1 ) = 600 / 500 = 1 and 1/5 or 1.2 %
So only 1.2% of a Gold Medal is actually Gold.
Olympic Games Improvement Percentages
The following website has some interesting statistics on the Percentage Improvements which have been made in various Olympic events through the use of Technology.
(Go to the end of the article to find the percentages).
http://www.azcentral.com/news/articles/2012/09/06/20120906olympics-new-performance-devices.html
Here are some interesting facts from this article.

Image Source: http://img05.beijing2008.cn
In the 100-meter sprint, Athletes’ performance improved 24 percent over the last 100 years, but only 4 percent is due to tighter aerodynamic clothing. The other 20 percent is a result of improvements in physiology, nutrition, coaching, better running tracks and other factors.

Image Source: https://c479107.ssl.cf2.rackcdn.com
In Cycling, technology has been the biggest factor in improvements: 101 percentage points of the 220 percent in improvement in the one-hour cycling record has been found to be due to developments in bike aerodynamics.

Image Source: http://graphics8.nytimes.com
In Pole Vaulting, Poles went from wood to bamboo and then to metal before switching to carbon or glass fiber. It is believed that 27 percent of the 86 percent improvement in Pole Vole Height achievements has been due to these changes in materials.
Latest Shoe Technology

Image Source: http://www.ecouterre.com
London 2012 will see the debut of Nike’s new minimalist marathon running shoe called the “Flyknit”.
This shoe is a virtually seamless meshlike shoe, that is in keeping with the barefoot “less-is-more” approach to running.
The 5.6-ounce weight is 19 percent lighter than the Nike shoes worn by marathoners in the men’s 2011 World Championships.
Adidas is introducing a 3.5-ounce spiked sprinting shoe, called the “Adizero Prime SP”.
These lightweight shoes, worn by U.S. sprinter Tyson Gay, are based on the assertion that lower mass allows runners to accelerate and change direction faster.
Plenty of Mathematics is involved with the design of these running shoes including Geometry, Symmetry, Distribution of Forces, Tensile Forces of Materials, as well as Energy and Impact Absorption.
Many Calculations and Algebra Formulas are also associated with the computer aided design, manufacture, and testing of this new technology footwear.
Information Source: http://www.azcentral.com/news/articles/2012/09/06/20120906olympics-new-performance-devices.html
Training Mathematics

Image Source: http://sp.life123.com
As part of their training program, all Olympic atheletes will probably do some regular workouts in the gym doing weight training.
The type of program they do is dependant on the events they are in.
Setting up a weight training program is in fact very mathematical, and involves the concept of “1RM”.
1RM is the maximum weight a person could lift to do one repetition of a given exercise.
Rather than actually attempt to load the weights up to do 1RM, which could result in muscle injury, there is a mathematical calculation that is performed, usually by an Online Calculator.
Once the 1RM value has been obtained for a given weight lifting exercise, Percentage mathematics is then used to work out the training program as per the following table:
The types of training indicated in the above table, as per the “Brian Mac” site are as follows:
Strength Endurance
The aim is to develop muscles that are able to to produce repeated contractions under conditions of fatigue. This requires high repetitions (15+) with light loading (30-50% of 1RM). Appropriate for field sports, rowing and martial arts.
Power
The aim is to develop fast powerful movements. This requires medium number of repetitions (6-10) with medium to heavy loading (70-80% of 1RM). Appropriate for power based events e.g. sprinting, jumping (long jump), throwing (Javelin).
Maximum Strength
The aim is to enable maximum loads to be lifted. This requires low number of repetitions (1-5) with heavy loads (80-100% of 1RM). Appropriate for Power Lifting, Olympic Lifting, Shot Putt.
In the table shown previously, all weight to be lifted is expressed as a percentage of the “1RM” weight value which is set at 100%.
The 1RM weight is the maximum weight we can lift for a specific exercise, where the weight is so heavy that we are only able to do one repetition of that weight. We are not strong enough to do a second lift of that weight.
As we train and get stronger, this 1RM value may become larger, and so it needs to be recalculated from time to time.
For full detaled information on the Mathematics of Weight Training, click the link below to view our complete lesson on this.
http://passyworldofmathematics.com/weight-training-mathematics/
Interactive Graph of Medal Counts

Image Source: http://infosthetics.com
The following link is to an interactive graph which shows how many medals countries have won at each Olympic Games.
The number of medals is shown by bubbles. The size of each bubble indicates how many medals were won by that country.
As you move your mouse onto a given bubble, it gives a count of the total Gold, Silver, and Bronze medals for that particular country.
Clicking on each year on the timeline, gives the graph of all countries medal counts for that particular Olympics.
We found that clicking the “By Ranking” tab gives the easiest graph to read, (Default is by Geography as shown above).
http://www.nytimes.com/interactive/2008/08/04/sports/olympics/20080804_MEDALCOUNT_MAP.html
Here is what a typical medals graph looks like in “By Ranking” view:

Image Source: http://infosthetics.com
This Interactive Medals Graph is great for having students gather data to graph the number of medals won by a given country over several years of Olympics, as well as Percentages of Gold Silver and Bronze medals comparisons, and several other statistical investigations.
Australian Dollar vs Gold Medals

Image Source: http://australianindependentbusinessmedia.com.au
Alan Kohler, an Australian Finance Specialist, has recently produced the above graph on his website:
According to Alan, this graph historically shows that whenever the Australian dollar took a fall compared to the US Dollar, Australia performed very well at the Olympics.
At the current time of the 2012 London Olympics, the Australian Dollar continues to rise with insignificant falls in the graph (the blue line).
According to Alan, this could mean that Australia will not win many gold medals at London.
However, here at Passy World, our interpretation of the graph is that when the Australian Dollar has been on the rise, then a surge in Gold Medals follows.
Therefore we believe that Australia could be up for winning quite a few gold medals at the current London Olympics.
The bottom line here is that there is no real world reason that winning Olympic Gold Medals should be related to the value of the Dollar.
The mathematical correlation between the two graphs shown above is interesting, but at the same time is probably not a true predictor of team performance.
Maths Behind the Olympic Games

Image Source: http://atlechic.webcindario.com
The following web page has some great information about Olympic events such as High Jump, where the athelete’s center of gravity is of critical importance to getting a good result.
http://motivate.maths.org/content/Olympics
Olympic Mathematics Activities

Image Source: http://www.dsgnwrld.com
The “Maths and Sport” Website has a great set of activities that students can do in class which are related to the Olympic Games.
There are also teacher guides and lesson plans for each activity.
The link to this University of Cambridge website is as follows:
http://sport.maths.org/content/
There are also Olympic Games Maths Activities at the following links:
http://www.suffolkmaths.co.uk/pages/Maths%20Projects/Olympic2012.htm
http://www.tes.co.uk/article.aspx?storyCode=6170892
http://www.tes.co.uk/TaxonomySearchResults.aspx?parametrics=primary,42198,43720,EVT-4202&mode=browse
If you enjoyed this article, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.
Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.
Enjoy,
Passy