
Image Source: http://www.soulwire.co.uk
Triangles can even be used in Art, as shown above in the great piece of artwork by Justin Windle from Soulwire.
What we really like about this picture is the clever shading of the triangles to create various 3D effects throughout the drawing.
Triangles are also used in GPS Location and Navigation.
Any position on Earth that is beamed to three or more GPS Satellites creates unique distances which form triangles of known sizes.

Image Source: http://www.cdxetextbook.com
The mathematical process to work out the actual GPS location on earth uses “Triangulation” or “Trilateration”.
Basically there are 24 GPS satellites orbiting the earth in fixed positions, so that any GPS unit will always be able to connect to at least three of the satellites at any time of the day or night.
From these radio frequency connections, many triangles are formed, calculations performed, and the latitude, longtitude, and elevation, of the position on earth is determined.
The actual mathematics is quite complicated, and uses 3D triangles, which form cone like Pyramids, and this is called “Trilateration”.

Image Source: http://www.technogps.com
For an excellent overview of exactly how GPS works, check out the following web page:
http://www.cdxetextbook.com/auxil/periph/satellite/triangulation.html
In this lesson, we are not looking at the details of GPS Triangles, but rather the Exterior Angles which are formed when we extend the sides of any Triangle.
Exterior Angles can be used as part of GPS Calculations, but this mathematics is quite a bit more advanced than the basics we need to cover here.
Exterior Angles of a Triangle
The Exterior Angles of a Triangle are formed by extending the sides of the Triangle, so that a 180 degree Supplementary Angle straight line is formed.
This is shown in the following diagram.

Image Copyright 2012 by Passy’s World
Every Triangle has three Exterior Angles that match up with each of the three Interior Angles.
Each Exterior Angle sums with its adjacent Interior Angle to form a 180 degree straight line.
The following example shows how we extend a typical triangle’s sides to create its three Exterior Angles.
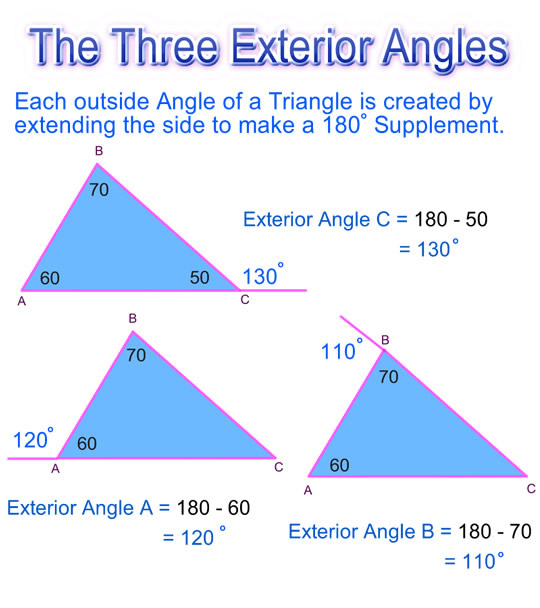
Image Copyright 2012 by Passy’s World
Exterior Angle Theorem
Based on the fact that the Interior Angles of all triangles add up to 180 degrees, and that the Exterior Angle and its partner angle also always add to 180 degrees, Mathematicians have been able to develop the rule shown in the diagram below.
They call this rule a “Theorem”, which is just a fancy name for any shortcut rule we can use in Maths.
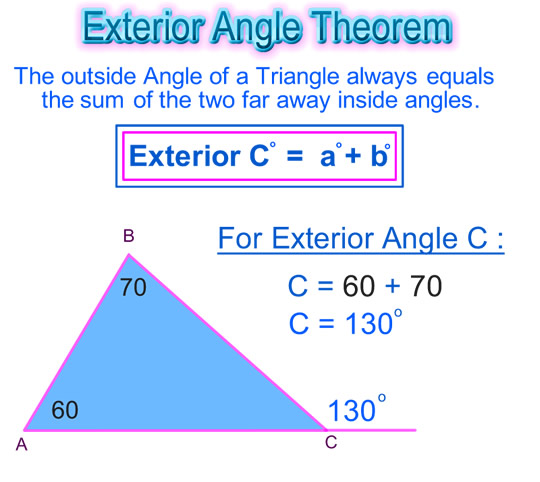
Image Copyright 2012 by Passy’s World
Exterior Angle of Triangle Examples
In this first example, we use the Exterior Angle Theorem to add together two remote interior angles and thereby find the unknown Exterior Angle.
This is the simplest type of Exterior Angles maths question.
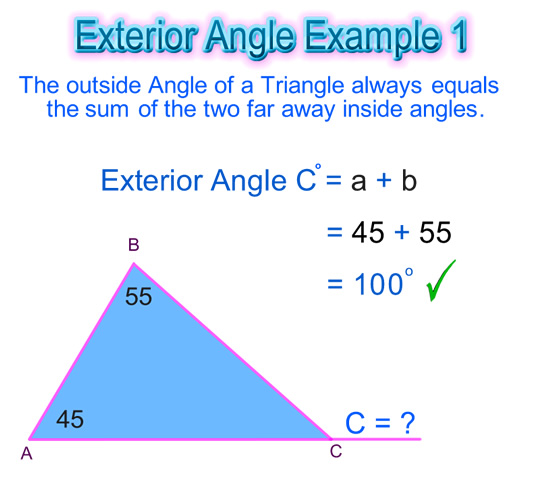
Image Copyright 2012 by Passy’s World
In this next example we find the missing Interior Angle by using the Exterior Angle Theorem.

Image Copyright 2012 by Passy’s World
This final example shows an Exterior Angle question that requires the setting up and solving of Algebra Equations.
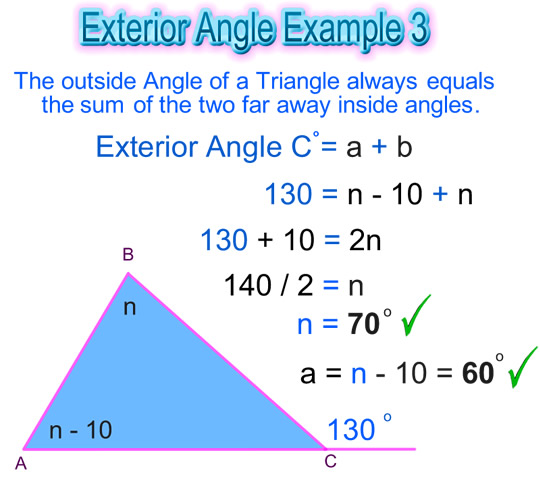
Image Copyright 2012 by Passy’s World
Videos About Exterior Angles
The following video from YouTube shows how we use the Exterior Angle Theorem to find unknown angles.
Here is another video which shows how to do typical Exterior Angle questions for triangles.
This video shows some examples that require algebra equations to solve for missing angle values.
Exterior Angle of a Triangle Worksheets
The links below are to web pages which have a range of questions involving exterior angles.
http://www.mathwarehouse.com/classroom/worksheets/triangles/remote-exterior-interior-angles.pdf
Related Items
Classifying Triangles
Angle Sum in a Triangle
GeoGebra
Interactives at Mathwarehouse
Jobs that use Geometry
If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.
Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.
Enjoy,
Passy



Pingback: Angles and Parallel Lines | Passy's World of Mathematics
Pingback: Isometric Drawing and 3D Cubes | Passy's World of Mathematics
Pingback: Geometry in the Animal Kingdom | Passy's World of Mathematics
Pingback: Jobs With Geometry | Passy's World of Mathematics