Mathematics of Aircraft Disasters

Image Source: http://www.aviationwa.org.au

If you don’t think Mathematics is vitally important, then think again!

The following news article we saw on an Australian news website today. It is all about a mathematical mistake that made a passenger plane nearly “stall” and fall out of the sky. It was very lucky that the plane did not crash.

In 2010, Pilots flying a regional Australian Qantas aircraft carrying 97 passengers were warned the plane was in danger of dropping from the sky during two bungled landing attempts.

An incident report released on February 9th 2012, said the captain and co-pilot wrestled with shaking joysticks as they tried to land the pitching Boeing 717-200 at an airport in Kalgoorlie, en route from Perth, in October 2010.

The Australian Transport Safety Bureau report found the drama resulted from the captain entering the wrong data on the plane’s weight into the flight computers — an error unnoticed by the co-pilot.

The plane’s landing weight was being calculated at 9.4 tonnes LIGHTER than its actual weight. (9.4 tonnes is about the weight of nine motor cars, and so this represents a very serious error).

This meant the subsequent mathematical calculations performed by the in-flight computer made the plane approach the landing strip at the wrong speed and angle.

The botched calculations twice triggered the “stick shaker” which alerts pilots to an impending aerodynamic stall — when the plane is no longer stable and may fall mid-air.

The warning was triggered during the first attempted landing at an altitude of 335 metres and again during the second attempt at 106 metres.

On both occasions the pilots failed to identify the underlying error and assumed air turbulence was to blame.

Normal descents take place at a constant airspeed and constant angle of descent (3 degree final approach at most airports). The pilot controls the angle of descent by varying engine power and pitch angle (lowering the nose) to keep the airspeed constant. If the nose is too high for the chosen power the airspeed will decrease until eventually the aircraft stalls or loses lift. 

Landing is the most difficult part of a flight, and mathematical equations programmed into the flight computers are used to instruct the pilots on how to safely guide the plane to the airport runway. Based on continuous mathematical calculations using equations and measurements made by the plane’s instruments, these computers give emergency warnings when things are not going correctly. (This part of the plane is called the “Avionics”). 

Because the plane’s computer thought the plane was lighter than it actually was, it calculated an angle of descent and speed that were not powerful enough for the heavy plane. The plane’s avionics computer detected that the plane was not going fast enough to maintain lift under its wings, and was in danger of stalling. This would mean the plane was coming down way too fast, and was going to land short of the runway, so the pilots had to quickly abort the landing.

Investigators said a lack of standard cross-checking routines allowed the data error to go unnoticed. They also said the captain, although “well rested”, had struggled to manage his fatigue owing to numerous roster changes.

The plane was operated by Cobham Aviation Services but was flying under the banner of QantasLink.

Raw Information Source: http://news.ninemsn.com.au/national/8417234/qantas-pilots-warned-plane-may-fall-from-sky

Overloaded Concorde Take Off Crash

Image Source: http://lh3.ggpht.com

Air France Flight 4590 was a Concorde flight operated by Air France which was scheduled to run from Charles de Gaulle International Airport near Paris, to John F. Kennedy International Airport in New York City.

On 25 July 2000, it crashed in Gonesse, France. All one hundred passengers and nine crew members on board the flight died. On the ground, four people were killed with one left injured.

This was Concorde’s only accident in 25 years of flying in which fatalities occurred.

Post-accident investigation revealed that the aircraft was exceeding the maximum weight for ambient temperature and other conditions, and up to one ton over maximum structural weight.

Also, as it left the gate, it was loaded such that the centre of gravity was excessively located towards the back of the plane.

Although the overloaded weight did not directly cause the crash, it is thought to be a contibuting factor. The weight and incorrect centre of gravity probably helped tilt the plane upwards at an angle too large which caused fatal “stalling”.

“Stalling” is when a big plane is pointing up or down at an angle where it stops getting lift from air flowing under its wings. The plane then tilts up and literally falls backwards out of the sky, or goes into an uncontrollable nose dive.

Read full details of the crash on Wikipedia by clicking the link below:

http://en.wikipedia.org/wiki/Air_France_Flight_4590

Here is a diagram showing how the crash happened.

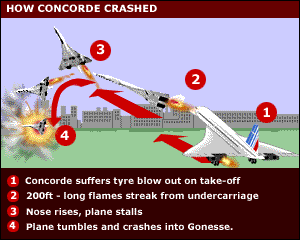
Image Source: http://4.bp.blogspot.com

The following four minute video describes the Concorde plane, one of the most beautiful passenger planes to ever operate, as well as the fatal Flight 4590 crash.

[youtube http://www.youtube.com/watch?v=0-een7na2GU]

Overloaded Runaway Train

Image Source: http://www.gordon-elias.com

It is important that the weight of freight trains is accurately known when planning their trips.

On May 12th, 1989 the San Bernardino train disaster occurred in San Bernardino, California.

An out of control Southern Pacific Railroad freight train derailed on Duffy Street on the very steep Cajon Pass, killing two crew members and two children, ages 7 and 9.

Eleven homes were severely damaged or completely destroyed in the accident.

Thirteen days later fuel leaking from a pipe line damaged in the accident ignited, killing another 2 people and causing further damage to homes.

The cause of the crash was found to be that the weight of the train had not been correctly calculated. As a result there were not enough engines with enough braking power hitched up to the train for its steep descent down to San Bernadino from the nearby mountains.

Information Source: http://crazycrashes.wordpress.com/train-wreck-historical-timeline-1950-to-2000/

The following video is a 45 minute National Geographic documentary on the San Bernadino disaster.

The first couple of minutes of the video summarise what happened.

[youtube http://www.youtube.com/watch?v=OhOByrKb6FY]

Conclusion

Even though we have computers to do all the complicated mathematics involved with large transport machines, these computers still need to be given accurate numerical input including total weight, and other relevant variables such as wind direction and speed as well as runway surface conditions.

So next time they weigh your suitcase at the Airport, be confident that all the cargo and fuel gets weighed and added up correctly and entered into the plane’s flight computer.

Don’t worry, it is extremely unlikely that things will go wrong. Statistically planes are by far the most safest form of travel on earth.

Various estimates we found on the web rate the chance of dying in a plane crash to be about 1 in 9 milllion.

Information we found on the web says that the American National Safety Council puts your one-year odds of dying in a car accident at about one in 6500.

This means it is about 1400 times safer to fly in a plane than travel in a car!

The odds of dying in a car accident on the way to the airport, greatly surpass the odds of ever dying in a plane crash! (Because the Mathematics says so).

Related Items

AFL Ground Sizes
AFL Football GPS
AFL Football Statistics
Drag Racing Integers
eBay Math Problem
Interesting Circles
Interesting Percentages
Jobs that use Geometry
Math in Music – Fibonacci Sequence
My Virtual Home – Area and Perimeter
Off Road Motorcycling Algebra and Statistics
Real World Integers
Real World Straight Line Graphs I
Real World Straight Line Graphs II
Real World Charts and Graphs
Tall Buildings
Tsunami Mathematics
Weight Training and Percentages

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Enjoy,
Passy