
Image Source: http://www.seanasmith.com
Cable trains are called “Funiculars”, and are used to go up and down very steep incline Gradients.
These gradients are too steep to have a train push or pull the carriages, and so the carriages are pulled up and down by steel cables.
The steepest Gradient cable train in the world is actually right here in Australia, at Scenic World in the Blue Mountains.
The train runs at an angle of 52 degrees, which is steeper than any normal train or road vehicle could ever go.
Here is a great two minute video about the Australian Cable train at the Blue Mountains.
Definition of Gradient
Gradient represents how steep a slope is in terms of its vertical change over a given horizontal Distance.
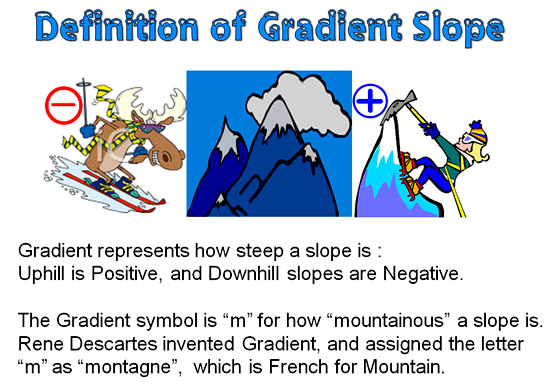
Image Copyright 2013 by Passy’s World of Mathematics
The Gradient is expressed as the Rise / Run
or as m = Vertical Change / Horizontal Change
A Gradient of m = 3/2 means that for every two meters we go horizontally, our hill goes up by 3 meters.
The Angle of Incline can be found by doing Inverse Tan-1 (3/2) which comes out as 56 degrees.
56 Degrees, or 3/2 is quite a Steep Gradient.
Fenicular Trains

Image Source: http://u.slimg.com
Many Feniculars are powered by large diesel or electric motors, but the Stanserhorn Funicular in Switzerland is “self-powered” and requires no energy.
It is the perfect eco-friendly form of naturally powered transport.
How the Swiss train works under its own power is by having a continuous cable connecting a carriage going up with a carriage going down.
When the “down carriage” is at the bottom of the mountain, the “up carriage” is at its stopping point further up the mountain.
The force of Gravity on the train carriage going down the mountain, pulls the other train carriage that is going up the mountain.
Along the way there are passing sections.
These are located at a midpoint where it is exactly known the up train will meet the down train.

Image Source: http://www.funimag.com
Here is a photo of the two cable trains passing each other.
Image Source: http://imagebanana.com
There are also “Funicular” self powered gradient cable trains in other parts of the world, such as the funicular which was built in 2005 along the Zambezi River, and is called the “Victoria Falls Eco-Lift”.

Image Source: http://www.funimag.com
If you are interested in Feniculars, then check out the following website, which has lots of Feniculars featured from all around the world:
Mountain Cable Car Gondolas

Image Source: http://4.bp.blogspot.com
On the Stanserhorn Mountain, the Fenicular Train can only go so far up the Mountain.
At the train’s finishing point, the gradient of the mountain slope is too big for safe train travel.
Passengers get off the train, and board a cable car which goes to the top of the mountain.
By stringing the Gondolas of the Cable Car above the ground from towers of varying heights, the slope of the cables can be kept at an approximate 45 degree value where the cable cars can operate.

Image Source: http://flickr.com
Stanserhorn Videos
Here is a video which shows the Stanserhorn Fenicular journey.
It is a long eleven minute video which shows the whole trip.
We suggest watching the 5 mins to 5 mins 30 secs part where the two trains meet; and then watch from 9 minutes 20 seconds to 10 minutes 30 seconds part where a train comes into the mid mountain station to meet up with the cable car.
This second video shows the cable car ride section of the journey to the top of the mountain.
Be warned that it does have an interesting swiss folk song musical backing track.
Finally, if you are mega-fit, you can enter the race to the top of the Stanserhorn, which is shown in the following video.
The Race is conducted at the height of summer, when there is no snow on the mountain.
Finally, here is a good five minute video about why trains have trouble going up any type of hill.
Mountain Vegetation and Temperature Gradients
The air temperature is warm at the base of the mountain with the sun shining and blue sky, but very cold with ice and snow at the top of the mountain.
There is a decreasing gradient as we go up the mountain with temperature progressively getting lower.
The Vegetation and Soil also has a Gradient Change as we go up the mountain.
The vegetation and soil decreases follows along with the temperature decrease as we go up the mountain.
In the lower section where the train starts, there are houses, grasslands, flowers, and deciduous trees.
There is plenty of rich soil, and lush pastures for cows and other livestock.

Image Source: http://amazonnaws.com
However, as we ascend the mountain, the Gradient becomes steeper, there is less vegetation, with rocks amongst the grass, and only evergreen pine trees.
The vegetation change is due to the decrease in temperature, and the increase in rockiness, (less soil and more rock and gravel), as we go higher up the mountain.

Image Source: http://www.funimag.com
As we get right up near the top of the mountain, the sides are very steep, there is virtually no soil, and the only plants which can survive in the cold snow are pine trees.

Image Source: http://tripadvisor.com
As we get close to the top where it is very cold, only a few pine trees manage to cling to the steep sides of the mountain.

Image Source: http://tripadvisor.com
The top of the mountain has a great view of the Swiss Alps, but the temperature is a lot colder than where the sun is shining on the lake at the base of the mountain.

Image Source: http://tripadvisor.com
Passy has been up the Stanserhorn Mountain in Switzerland, and it is a unique gradient experience which is highly recommended!
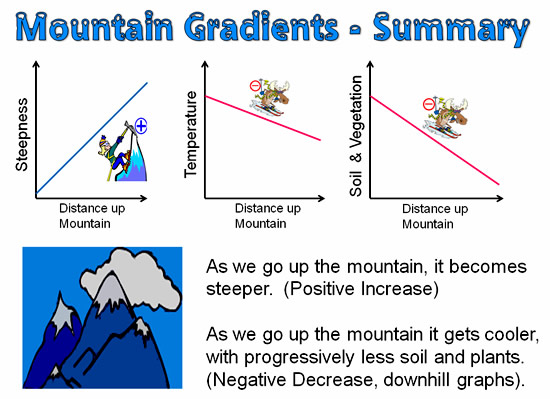
Image Copyright 2013 by Passy’s World of Mathematics
Ascending any big mountain, there are the gradients of the mountain slopes, the temperature gradient, and the soil and vegetation gradients.
Changes in these real world gradients make for a unique and scenic journey from the lakeside town of Luzern, up to one of the highest peaks in Switzerland.
Related Items
The Cartesian Plane
Plotting Graphs from Horizontal Values Tables
Plotting a Linear Graph using a Rule Equation
Plotting Graphs from T-Tables of Values
Finding Linear Rules
Distance Between Two Points
Real World Straight Line Graphs I
Real World Straight Line Graphs II
Subscribe
If you enjoyed this lesson, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our website, then please email us at the hotmail address shown in the right hand side bar of this page.
Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.
Like Us on Facebook
Our Facebook page has many additional items which are not posted to this website.
These include items of mathematical interest, funny math pictures and cartoons, as well as occassional glimpses into the personal life of “Passy”.
Check it out at the following link:
https://www.facebook.com/PassysWorldOfMathematics
While you are there, LIKE the page so you can receive our FB updates to your Facebook News Feed.
Help Passy’s World Grow
Each day Passy’s World provides hundreds of people with mathematics lessons free of charge.
Help us to maintain this free service and keep it growing.
Donate any amount from $2 upwards through PayPal by clicking the PayPal image below. Thank you!
PayPal does accept Credit Cards, but you will have to supply an email address and password so that PayPal can create a PayPal account for you to process the transaction through. There will be no processing fee charged to you by this action, as PayPal deducts a fee from your donation before it reaches Passy’s World.
Enjoy,
Passy




Pingback: Gradient and Slope | Passy's World of Mathematics
Pingback: Midpoint Between Two Points | Passy's World of Mathematics
Pingback: Gradient Slope Formula | Passy's World of Mathematics
Pingback: X and Y Intercepts | Passy's World of Mathematics
Pingback: Coordinates Bingo Game | Passy's World of Mathematics