
Image Source: http://plusmood.com
Basic Multiplication
The following example shows the simplest Algebraic Multiplication.
In this example we have to increase “3b” by a factor of 4.
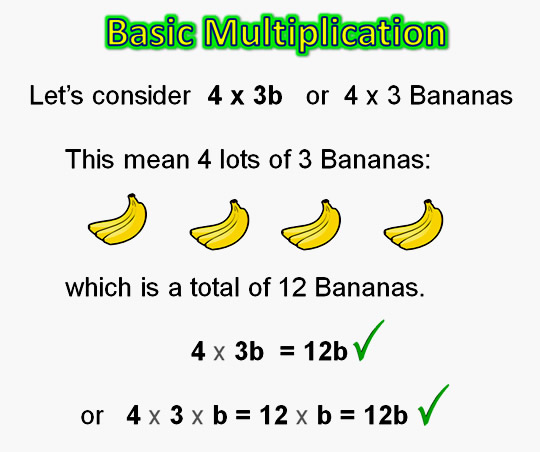
Image Copyright 2012 by Passy’s World
Algebra Multiplication
Now let’s consider the multiplication of 4a x 3b or 4 Apples x 3 Bananas
This is really strange to multiply Apples by Bananas because they are different items !
Let’s do it anyway ! First let’s put in all the multiply signs:
4 x a x 3 x b (Now adjust the order so the numbers come first)
In multiplying we are allowed to rearrange the order because it does not change the answer.
Eg. 5x2x3 = 30 and 2x5x3 = 30 and 5x3x2 = 30 and so on.
Mathematicians call this the “Commutative Property” : Factors can be multiplied in any order and the product is always the same.
So let us rearrange the order of our apples and bananas multiplying:
4 x a x 3 x b
= 4 x 3 x a x b (We can work out the number part as 4 x 3 =12)
= 12 x a x b
= 12ab (Remember in Algebra we leave out x signs from answers)
Now this is really hard to get your head around, but 12AB could be the number of Grapes that we have.
In this case we have lots of Grapes!
The Total Grapes = 12 x the number of Apples x the number of Bananas.
So 4a x 3b is an expanded way of saying that we have 12 x a x b or 12ab.
Practical Application of Algebra Multiplying

Image Source: http://www.xenian.com.au
Hopefully the following will help you understand one real world use of Algebra Multiplication.
Algebra multiplication equations are useful for Restaurants and the Hospitality Industry, where catering is done in large dining rooms.
It would be a major fail to set up a function room or restaurant and not have enough knives and forks for all of the guests to be able to eat!
Real World Example
For a function we might have “T” tables that each seat “N” people, where N could be 6, 8, 10, or 12 depending on the function room.
Each person at a table requires two big forks, (one for eating the entree and one for the main course).
The total number of forks required for setting all the tables at the function is:
F = 2 x N x T = 2NT
In the good old days, Algebra Calculations like this would be worked out on paper, but these days there is probably a mobile device or computer app which can do most of the work for us.
We would simply enter:
Number of Tables
Maximum Number of people at each table
Number of big forks needed per person
Number of little forks needed per person
Number of big knives needed per person
Number of little knives needed per person
Number of spoons needed per person
Number of teaspoons needed per person
and the App would calculate our total cutlery requirements.
The Algebra is programmed into the App by the person who wrote the App.
Algebra is essential for programming any computer device, and a valuable skill to have in these modern times.
Steps for Algebra Multiplication
The steps for carrying out Basic Algebra Multiplication are as follows.

Image Copyright 2012 by Passy’s World
Integers Multiplying Rules
For Multiplying integers, we use the following set of rules.

Image Copyright 2012 by Passy’s World
If you need to review Multiplying Integers, then click the following link:
http://passyworldofmathematics.com/multiplying-integers/
Also it is important to remember for this lesson that:
b is the same as 1b, and -k is the same as -1k etc
Scope of This Lesson
Algebra Multiplication can involve Exponents, Indices, Powers, and multiple terms inside brackets.
The lesson we are doing here is an introduction to Algebra Multiplication and only covers beginner’s basics.
Our examples will only be for very simple “monomials” and “terms”.
More advanced Algebraic Multiplication involving Powers and Brackets will be covered in separate lessons.
Algebra Multiplying Examples
The following examples show how to do some typical Algebraic Multiplication and simplification questions.
It is important to remember that for negative Variable letters without coefficients, we do the following:
-y is the same as -1y, so we re-write -y as -1y when multiplying
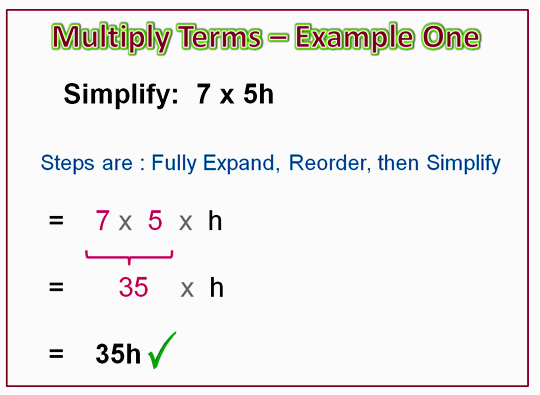
Image Copyright 2012 by Passy’s World

Image Copyright 2012 by Passy’s World

Image Copyright 2012 by Passy’s World

Image Copyright 2012 by Passy’s World
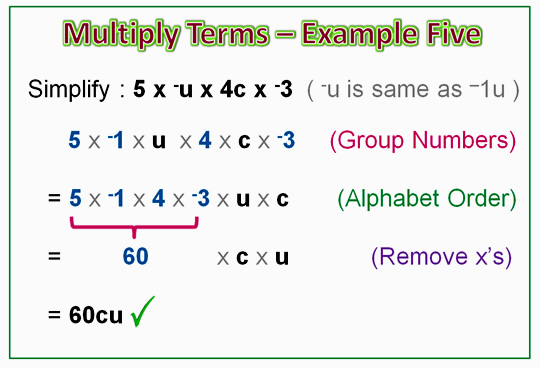
Image Copyright 2012 by Passy’s World
Related Items
Algebra Expressions
Algebra Terms Coefficients Variables and Constants.
Algebra Substitution Using Positive Numbers.
Algebra Substitution Using Negative Numbers.
Basic Powers Exponents and Indices.
Substitution Using Powers Exponents and Indices.
Identifying and Combining Like Terms.
Real World Algebra Formulas
Survivor Algebra – Class Activity
If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.
Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.
To find out exactly how free subscription works, click the following link:
If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown below.
If you are a subscriber to Passy’s World of Mathematics, and would like to receive a free PowerPoint version of this lesson valued at $4.99, but 100% free to you as a Subscriber, then email us at the following address:
Please state in your email that you wish to obtain the free subscriber copy of the “Basic Algebra Multiplications” PowerPoint.
Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.
Enjoy,
Passy





Pingback: Multiplying Algebra Exponents | Passy's World of Mathematics
Pingback: Algebra Dividing and Exponents | Passy's World of Mathematics
Pingback: Expanding Brackets Using Distributive Rule | Passy's World of Mathematics