Passy's World of Mathematics

Coffee Spill Mathematics

Posted on August 21, 2012 by Passy

Image Source: WordPress.com

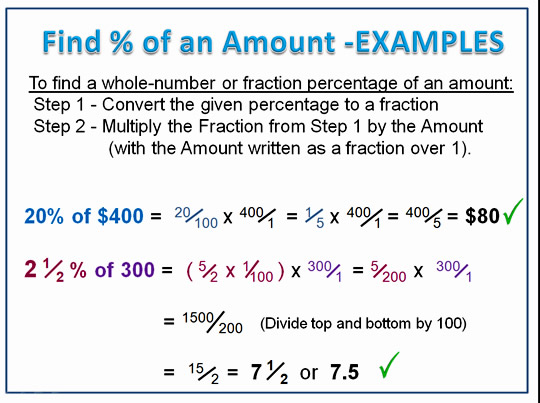
There are mathematical equations for just about everything these days: there is even mathematics for carrying cups of coffee!

A mathematical study has revealed that people walking with full cups of coffee will most often spill them at some point between the seventh and tenth step.

It just so happens that the human stride has almost the perfect frequency to drive the natural oscillations of coffee, when the fluid is in a typically sized coffee mug.

Two engineers, Rouslan Krechetnikov and Hans Mayer, set up an experiment: they asked people to walk at different speeds along a straight path with a filled coffee mug in hand.

The volunteers did this in one of two ways:

1) Focusing on the coffee mug and

2) Looking straight ahead.

A camera recorded each person’s motion and the mug’s trajectory, while a tiny sensor on the mug recorded the instant of spillage.

A fluid’s back-and-forth movement has a certain natural frequency, and this is determined by the size of its container. In their paper published last week in Physical Review E, Krechetnikov and Mayer show that everyday mug sizes produce natural frequencies that just happen to match those of a person’s leg movements during walking. This means that walking alone, without any other interference, is tuned to drive coffee to oscillate in a mug. But the researchers also found that even small irregularities in a person’s walking are important: These amplify the wilder oscillations, or sloshing, which bumps up the chance of a spillage.

The following video form ABC Catalyst summarises this experiment.

Watch it online at the following link:

http://www.abc.net.au/catalyst/stories/3568949.htm

Preventing Spillage

The researchers found that when study participants focused on their cups, the average number of steps they took before spilling coffee increased greatly.

Krechetnikov and his graduate student Hans Mayer, the primary author of the study, suggested two explanations for this result.

First, focusing on one’s cup tends to result in slower walking, and second, it dampens the noise, or chaotic sloshing, in the cup.

Secondly, a focused carry decreases the amount of noise because we perform “targeted suppression”, automatically counteracting the sloshing of the liquid with small flicks of our wrists, or because we simply hold the cup more steadily when we’re looking at it, the researchers could not say.

Third, accelerate gradually. If you take off suddenly, a huge coffee wave will build up almost instantly, and it will crash over the rim after just a few steps.

Concluding their study, the two engineers had some advice for coffee drinkers. They said leaving a large gap between the coffee and the top of the drinking vessel, and walking slower, prevents spillages.

They added that watching the mug, rather than the floor, while carrying it proved to be a more effective coffee-holding method.

So to prevent coffee spills do these three things:

a) Slow down

b) Watch your coffee, not what’s ahead of you

c) Don’t fill your cup too high

In regard to c), “too high” actually has a mathematical formula: keep your coffee level at least one-eighth of the cup’s rim diameter from the top and you will never spill your coffee!

Liquid Sloshing Dynamics

Image Source: http://www.ascience.com

The area of Science, Engineering, and Mathematics which studies the back and forth movement of liquides is called: “Liquid Sloshing Dynamics”.

Liquid Sloshing engineering studies, were first done to stabilize fuel tanks inside missiles, rockets, and satellites.

Quite complicated Mathematics and Equations are used in these studies.

Image Source: Slosh Formulas from ABC Catalyst Video

The mathematical equations have been programmed into computers to creates sloshing simulators.

Image Source: http://www.ascience.com

For more details about sloshing simulation, go to the followining link:

http://www.ascience.com/03152006Slosh.htm

The problem of liquid sloshing in moving or stationary containers is an onging concern for aerospace, civil, and nuclear engineers; mathematicians, physicists and designers of road and ship tankers.

Engineers already know of slosh-control techniques: Tanker trucks contain inner ridges, or baffles, to damp the liquid’s motion, because too much sloshing can make a truck overturn.

Image Source: http://wordpress.com

Preventing Sloshing in Tanker Trucks

Image Source: http://www.rapidspray.com.au

An Australian company specialising in Slosh Engineering is “Rapid Spray. The photo above shows them testing slosh on Water and Weed Spraying Tanks.

Rather than building fence type ridges inside the truck tank, Rapid Spray obtain stability by filling the tank with hollow “Baffle Balls”.

These balls slow down the movement of liquid in the tank, and stop large sloshing which could tip the truck over.

Image Source: http://www.rapidspray.com.au

The more traditional method of stopping excessive sloshing in tankers, involve placing baffle dividers in the tanker as shown in the following diagram.

Image Source: http://www.nomenclaturo.com

Note that there is also considerable mathematics and engineering involved with the braking systems of tanker trucks.

As the tankers drop off loads and become less full, the brakes need to mathematically adjust for the lighter load, so that they do not come on full load force and lock up the wheels and skid the tyres.

Roller Hockey Application

Image Source: http://www.roller-hockey.co.uk

According to Wikipeadia, the effect of slosh is used to limit the bounce of a roller hockey ball.

Water slosh can significantly reduce the rebound height of a ball, making it stay low and behave more like a hockey puc than a ball.

Many of the balls for roller hockey commonly contain water to reduce their bounce height.

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.

Enjoy,
Passy

Posted in Algebra, Math in the Real World | Tagged algebra equation, cofee spill math, coffee spill mathematics, fluid dynamics, fluid sloshing mathematics, how sloshing works, math equations, real world algebra, real world equation, real world mathematics, sloshing mathematics | 1 Comment

Percentage Discounts

Posted on August 3, 2012 by Passy

Image Source: http://www.dobi.nu

The above TShirts were made by the very talented designer Rob Dobi.
You can buy Rob’s TShirts for around $20 each online at the following link : http://www.merchline.com/fullbleed/

Let’s say that our favorite clothes shop is having a 15% off sale on Rob Dobi TShirts. In the shop we see a really nice shirt that has a price tag marking the shirt’s normal retail price as $30.

In Mathematics, we call this normal retail price the “Marked Price” or “MP”.

Since the shirt is on sale at 15% off, we know that we are going to get a discount on the $30 Marked Price, and pay less than $30 for the shirt.

The amount we are taking off the normal Price is called the “Discount”.

The discount is how much money we are going to save if we buy the shirt during the sale, rather than buy it for the normal recommended retail price.

We can calculate the discount as follows:

Discount = 15% of Marked Price

(remember “%” means / 100 and “of” means multiply)

= (Discount % / 100) x Marked Price
= 15 / 100 x $30
= 15 divided by 100 x $30
= $4.50

So the dollar saving we will make during the Sale is $4.50

For calculating the Discount $, the Algebra formula is as follows:

Discount = (Discount% / 100) x MP (where MP = Marked Price)

The Price we actually pay for the shirt is called the “Selling Price”, “Sale Price”, or “SP”.

For calculating the Selling Price, the Algebra formula is as follows:

Selling Price = Marked Price – Discount

For our shirt the Selling Price is:

SP = MP – Discount
= $30 – $4.50
= $25.50

So we will pay $25.50 for our shirt, if we buy it on sale.

In this lesson we look at how to work out Percentage Discounts.

Percentage Discounts – Key Words

There are a number of special words that are used when we talk about selling items for profit.

These will become more familiar as we work through this lesson.

Here are the set of Key Words we need to know for dealing with Discounts.

Algebra Formulas for Discount Questions

To calculate Discount and Selling Price, we use the following mathematical formulas.

Selling Price (Multiplying Factor Method)

Image Source: http://resources0.news.com.au

Another way of determining the Selling Price is to use a method involving a “Multiplying Factor”.

The multiplying factor “M.F.” is a decimal value that we can multiply by the Marked Price to get the Selling Price.

In this method we use the following two formulas :

M.F. = (100% – Discount %) / 100

and then we calculate the SP using our M.F. answer like this:

SP = M.F. x MP

For our $30 shirt with a 15% Discount, the calculation is:

M.F. = (100% – Discount%) / 100

-> Substitute in our Discount% of 15

M.F. = (100 – 15) / 100
M.F. = 0.85

SP = M.F. x MP

-> Substitute in M.F.=0.85, and MP=$30

SP = 0.85 x $30
SP = $25.50

Percentage Discount or Percentage Saving

So far we have been looking at working out the Discount dollars for an item that is on sale, when we know what the Discount% value is.

Now let’s look at working backwards and finding the Discount% .

If we buy something on sale, then we can calculate the percentage we saved by doing the following steps.

The following example shows how to use these steps to do a Percentage Discount question.

Discount Percentage Example

Image Source: http://ilounge.com

Let’s say we go to a clearance store and they have a pair of Nike sports shoes we like, with a Marked Price on them of $110.

We really want to buy these shoes, but only we only have $90 to spend.

We tell the sales person we will offer them $80 for the shoes.

They talk to their manager and then tell us we can have the shoes for $90.

What is the percentage discount they have given us?

We need to follow the steps:

Work out Discount = Regular Marked Price – Sale Price
then
Divide by the Marked Price
then
Multiply by 100%

Discount = $110-$90 = $20

Next Divide by the MP which gives us: $20 / $110 = 0.181818

Now Multiply by 100% which give us: 0.181818 x 100% = 18.1818%

So we have managed to get an 18% Discount on the Shoes.

We can also represent this process by the following Algebra Formulas:

Dollar Discount = MP – SP

and

% Discount = (Dollar Discount / MP) x 100%

or combine everything into a single formula like this.

% Discount = ((MP – SP) / MP) x 100%

If you are using a Calculator to work out the answer, then putting in the brackets shown above is extremely important.

Let’s use the single formula to work on the $110 shoes being sold to us for $90.

For this example, MP = $110 and SP = $90

% Discount = ((MP – SP) / MP) x 100%

= (( 110 – 90) / 110) x 100

= (20 / 110) x100

On calculator do 20 divided by 110
and then multiply by 100

= 18.1818

= 18%

Discounts Summary

The following is a summary of the formulas we need to know for doing questions involving Percentage Discount.

Discounts Videos

The following video is by “YourTeacher.com”, and shows how to calculate a Discount and then work out the Selling Price.

This next video is by a mathematics teacher, and also shows how to how to calculate a Discount and then work out the Sale Price.

This final video covers everything we have done in this lesson.

In particular, six and a half minutes into the video, we see how to calculate the dollar discount as a percentage value.

Discount Calculator

We can use this calculator to get discounts. But this calculator is extra nice, because we can also enter the “Reduced” discounted price, and will it will calculate the original price.

So the calculator works in both directions.

Note that we always have to enter the Percent Value, but we only have to enter one of the three items on the top input line.

http://discount.miniwebapps.net/

Percentage Discount Game

This game teaches how to calculate percentages, as well as rounding off Answers. It is a UK game, and so money is in pounds and pence.

Click here to play Percentages Discount Game

Percent Discount – Discounts at Troy’s Toys

This game is quite challenging, and for some people they might need a calculator to divide the % value by 100, and then multiply by the dollar amount.

http://www.mathplayground.com/percent_shopping.html

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.

Enjoy,
Passy

Posted in Math Applications, Math in the Real World, Percentages | Tagged calculating discounts, calculating percentage amounts using decimals, calculating percentages, how to do discounts, how to do percentages, marked price, percent, percent discounts, percentage amount calculations, percentage amounts, percentage discounts, percentage mathematics, percentage of a number, precentage, sale price, what is selling price | Leave a comment

Finding Percentage Amounts Using Decimals

Posted on July 31, 2012 by Passy

Image Source: http://www.concordextra.co

The above 45 gram Chocolate Bar contains 25% Sugar.

How many Grams of Sugar are in this “Kit Kat” bar ?

Using Percentages, we know that Percent means /100, and so we can set up:

Grams of Sugar = ( 25 / 100 ) x 45 = 0.25 x 45 = 11.25 grams of Sugar.

Given that one teaspoon of granulated white sugar is equal to about 4.2 grams, our Kit Kat bar contains nearly three teaspoons of sugar.

Thanks to the Sugar, the chocolate bar tastes great, but each gram of sugar contains about 4 calories.

So to burn off the sugar in our Kit Kat bar we would need to burn off 4 x 11.25 = 45 calories.

In terms of Physical Exercise, a person of average weight would need to either skip for 3 minutes, or ride a bike at a fast speed for 3 minutes, or go jogging for 3 minutes to burn off these calories.

A great website for checking out how much sugar is in Food and Drinks is the following:

http://www.sugarstacks.com/

In this lesson we look at Finding the Percentage Amounts of items using Decimals and a Calculator.

The important thing to remember is that we are doing multiplication in these questions, and that “Percent” means divide by 100 or /100.

Steps for Finding Percentage Amount

To use Percentage to Find an Amount:

– Divide the Percentage by 100 to make a Decimal

– Multiply this Decimal by the Amount given

– Do NOT put a % sign on the answer.

Finding Percentage Amount Examples

The following examples show how to work out Percentage Amounts by first converting the Percentage to a Decimal.

Videos on Finding Amount Using Percentage

This video shows how to convert the % to a decimal, and then multiply by the number to obtain the answer.

Here is a video which shows some clever short cuts for calculating Percentage Amounts.

Percentage of a Number – Balloon Invaders

This game is like a Space Invaders type game to play, and is really fun.

Use the arrow keys to move the shooter, and space bar to shoot.

Click the link below to play this game.

http://www.mathplayground.com/balloon_invaders_percent.html

Percentages Mystery Game

This game is a movie mystery story with percentage puzzles along the way. Requires sound to hear the story.
Very well produced and entertaining.
Note that you need to mouse over area names on the map to find out how many squares they actually are.

Click the link below to play this game.

http://www.bbc.co.uk/bitesize/ks2/maths/number/percentages/play/popup.shtml

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.

Enjoy,
Passy

Posted in Percentages | Tagged calculating percentage amounts using decimals, calculating percentages, how to do percentage, how to do percentages, percent, percent math, percent maths, percentage amount calculations, percentage amounts, percentage mathematics, percentage of a number, precentage | 1 Comment

Percentage of Amount Using Fractions

Posted on July 28, 2012 by Passy

Image Source: http://cdn.sheknows.com

Let’s say we are making a Car Loan repayment, and we want to find the value of 10% of $200.

We are interested in doing this because our weekly Car Loan Payment is $200 plus 10% Interest.

Well 10% is one tenth,

and one tenth of 200 is the same as 200 divided by 10

which is 20.

So 10% of $200 = $20.

But what if we had the trickier task of getting 4 and 1/2 percent of $5000 ?

To work out this Percentage Amount, we need to convert our Percentage to a Fraction, and then multiply this Fraction by $5000.

If you do not remember how to convert a Percentage to a Fraction, then click the link below to do this lesson before proceeding any further.

http://passyworldofmathematics.com/converting-percentages-to-fractions/

Calculating Percentages of Amounts

In today’s lesson we are learning how to work out the Percentage amount or portion of a given numerical item.

The following is a summary of the steps required to do this.

To work out the % amount of a given value:

– Convert the % Value to a Fraction
( Put /100 or do x 1/100 )

– Multiply by the given amount Value

– Answer in correct units (Not % !)

Also remember these important things:

% means /100

/100 is the same as doing divided by 100 on a calculator

“of” means multiply

There is no percent sign on the final answer.

If we have a basic whole number Percentage given to us then the steps are simply:

Write the given % value as a fraction

then

Multiply this fraction by the given amount value

Note that the Amount Value needs to be written as a fraction over 1

Put appropriate units on the answer (do not put a % sign on it).

Percentage of Amount Videos

The following video shows how to work out a percentage of a number without using a calculator.

This next video shows a slightly different way of doing the same question.

This next video shows some handy “Mental Math” shortcuts for working out Percentages of Numbers and Amounts.

Percentage of Amount Examples

The following examples show how to do a simple whole number Percentage question, as well as a question involving fractions.

Online Percentage Calculator

The above is a dual purpose Percentages and Amounts Calculator

This calculator can be accessed at the following link:

http://www.onlineconversion.com/percentcalc.htm

It is easy to use and gives Percentages and Values as shown above.

Pick which calculation you want to do. You do not have to do both calculations and can just do one like we have in the above example.

Calculating Percentage Worksheets

“Homeschool Math” has a great free worksheet generator, (with answers provided).

We set up what we require on the following input table:

Then simply click the “Submit” button and our worksheet opens in a new window.

When you have completed the worksheet, simply click on “Answer Key” at the bottom of the screen to check your answers.

A new window will open with a worked answers sheet like this:

Click the link below to go to the Worksheet Generator.

http://www.homeschoolmath.net/worksheets/percent-of-number.php

Related Items

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.

Enjoy,
Passy

Posted in Percentages | Tagged basic percentage, calculating percentages, calculating percents, converting percentages, doing percentage, how to calculate percent, how to do percentage, how to find percentage, meaning of percent, meaning of percentage, percent, percent help, percent intro, percent math, percent maths, percentage, percentage help, percentage intro, percentage of a number, percentage of an amount, percentages and fractions, percents, working out percentages | 1 Comment

Complementary Percentages

Posted on July 27, 2012 by Passy

Image Source: http://wordpress.com

In Australia there are between 50 and 100 venomous species of Spider, but only two of these are life threatening to humans: the red-back and the Sydney funnelweb spider.

Based on this we could say that 3% of Ausralian Spiders are life threatenting to humans.

This means that 97% of Australian spiders are not life threatening.

The 97% figure was worked out by doing 100 – 3 = 97.

This is called using “Complementary Percentages”, and is the subject of this short lesson.

Complementary Percentages

Complementary Percentages add up to 100%, where 100% refers to the Grand Total amount.

30% and 70% are complementary percentages: (30 + 70 = 100) .

40% and 60% are complementary percentages: (40 + 60 = 100) .

20% and 80% are complementary percentagess: (80 + 20 = 100) .

Complementary Percentages are useful for finding out a missing percentage, if we know the other percentage.

For example if 70% of people like chocolate ice cream,
then 100 – 70 = 30% of people do not like chocolate ice cream.

If 60% of the students in a class are girls,
then we can work out that 100 – 60 = 40% of the class are boys.

If 43% of the population in India live in Urban areas,
then 100 – 43 = 57% of the population live outside of Urban areas.

The two percentages always have to add to 100.

So to find the missing percent, we do 100 – the percentage we know.

This can be a useful thing to remember when working out mathematics questions.

Complementary Percentages Examples

Image Source: http://mit.zenfs.com

According to Wikipedia, the United States of America is home to 4.52% of the earth’s population with more than 307,000,000 people. (as of July 17, 2010)

What percentage of the world’s population lives outside of the USA ?

We can use Complementary Percentages to work this out, because people either live in the USA or not in the USA.

Percentage of People not living in the USA = 100 – 4.52 = 95.48%.

Image Source: http://www.howitoo.com

If 80% of a group of 40 students passed their driving license test on the first attempt, then how many students failed the test ?

We know that 80% passed, so 100 – 80 = 20% did not pass because they failed.

20% of 40 = 20 divided by 100 x 40

= 8 students failed their driving test.

Image Source: http://eoimages.gsfc.nasa.gov

The idea of percentages adding to 100 in total, is not just limited to having two items.

Air in the earth’s atmosphere typically contains 78% nitrogen, 20% oxygen, 1% water vapour, 0.93% argon and 0.04% carbon dioxide.

The rest is made up of other gases.

What is the percent of other gases?

We can work this out by doing :

100 – 78 – 20 – 1 – 0.93 – 0.04

= 0.03% other gases.

Related Items

If you enjoyed this post, why not get a free subscription to our website.
You can then receive notifications of new pages directly to your email address.

Go to the subscribe area on the right hand sidebar, fill in your email address and then click the “Subscribe” button.

To find out exactly how free subscription works, click the following link:

How Free Subscription Works

If you would like to submit an idea for an article, or be a guest writer on our blog, then please email us at the hotmail address shown in the right hand side bar of this page.

Feel free to link to any of our Lessons, share them on social networking sites, or use them on Learning Management Systems in Schools.

Enjoy,
Passy

Posted in Uncategorized | Tagged Adding Percentages, complementary percent, Complementary Percentages, complementary percents, percent totals, percentage totals, percentages, Solving Percentages | 2 Comments

Passy's World of Mathematics

Coffee Spill Mathematics

Percentage Discounts

Finding Percentage Amounts Using Decimals

Percentage of Amount Using Fractions

Complementary Percentages

Translate Website

Popular Lessons

Latest Lessons

Our Facebook Page

Categories

Who has visited Map

Linking to Us

Visitor Statistics

User Feedback

Meta

Donate to Passy’s World

Like us on Facebook